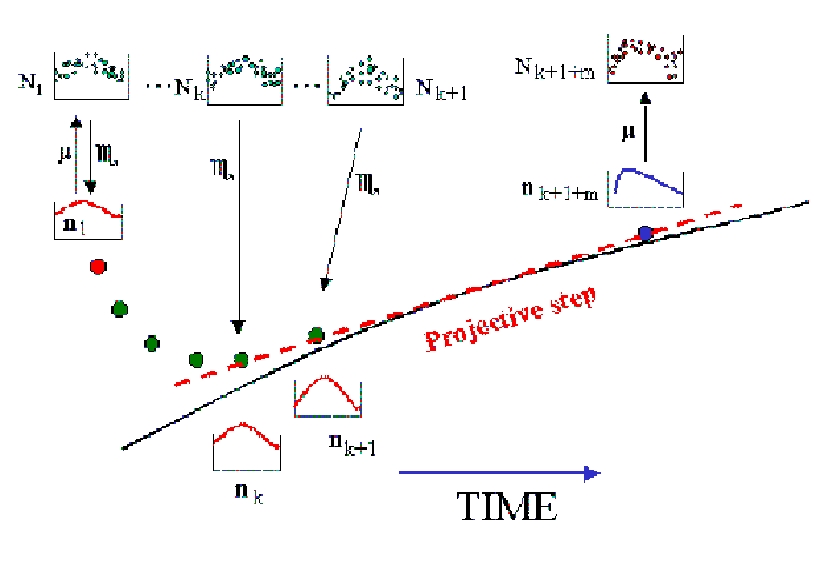
We illustrate the ``equation-free'' and projective integration approaches with the schematic in the figure above. We start with data from a coarse representation n<sub>1</sub> of the system. In the case of plasma physics this might correspond to data from a MHD representation of the plasma. This data is then ``lifted'' to a ``fine'' or kinetic representation N<sub>1</sub> whose moments correspond to the coarse representation. The kinetic representation could be a full particle model that could be evolved with the p3d code or a gyrokinetic representation that could be evolved with GS2. The kinetic model is now evolved k time steps forward with the fine scale integrator. These k time steps allow the kinetic system to relax to the ``slow manifold'' that governs the long time evolution of the system. This relaxation procedure is required because the initial state will not generally be in a equilibrium in the kinetic sense.

After k time steps, the data from the kinetic model N_k is now ``restricted'' onto the coarse representation n<sub>k</sub>. In the mean time the kinetic model is further stepped by one or more time steps and this data is then ``restricted'' onto a second coarse representation n_k+1. The coarse representations n_k and n_k+1 provide the basis for projecting forward the coarse representation with a large ``projective'' time step along the ``slow manifold'' to n_k+1+m. The process then repeats. It is important to note that the projection forward of the coarse representation did not require any knowledge of an equation governing the dynamics of the coarse representation -- hence the name ``equation-free'' denoting this technique. Further refinements of this approach include producing an ensemble of fine scale representations. This leads to more rapid convergence to the ``slow manifold'' than simply extending the time integration of the fine representation.