|
Abstract:
In this talk show that there exists no self-similar
finite time blowing up solution to the 3D
incompressible Euler equations if the vorticity
decays or grows sufficiently fast near infinity in
 .
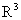We also rule out the possibilities of asymptotically
self-similar singularities for both of the 3D Euler
and the 3D Navier-Stokes equations.
[LECTURE SLIDES]
|
